Concrete abstractions
Spaces of observation
Space begins by tracing signs on a blank page.

A series of numbers climb the left margin, one on top of the other.

Another series, less dense, just long enough to establish scale and direction, appears at the bottom of the page, from left to right.
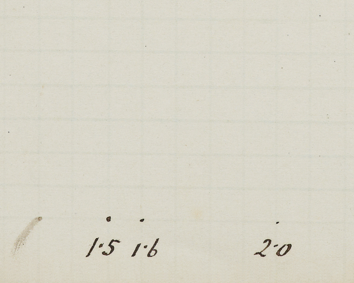
More numbers, and an equal quantity of dots, have been placed around the centre of the grid. Unlike the first two series, they are irregularly distributed.

The numbers on the left represent quantities. At the bottom are prices. The unruly ones, years. Perhaps, the whole graph, drawn by William Stanley Jevons in 1865, represents the demand for coal.

This is the year 1857.

This one is 1859.

This is 1860.

This is 1858.

This is 1863.

This is 1862.

This is 1861.

And this is 1865.

Later, almost as an afterthought, a dashed line is drawn.

It does not represent anything.

Yet its focus and regularity appear to call the dots to order,

to a regular function.

Suddenly, the isolated dots look confused, disorganized, almost stupid.

From political economy to economics
The drawing was produced by the nineteenth-century political economist William Stanley Jevons. It is one of the earliest known attempts to derive a mathematical function from plotting statistical data on prices and quantities.
In the graph, we can recognize the function by the dashed line.
It is interesting that the dashes do not connect the dots, which would have provided an irregular line; rather, the dashes form a smooth curve, indicating where Jevons thought the dots would have gone had the observations been recorded more accurately.
A smooth curve assumes that the relationship between the two variables – the historical price of coal and the quantity of coal sold at that price – are directly correlated.
It was at the end of the nineteenth century, when political economists stopped gaping at individual dots and started looking for patterns in the data, for ‘social facts’, that their field of study gave birth to economics, or the science of economy.
Today, even a cursory comparison of economics with other social and natural sciences reveals the unusual emphasis economists place on the use of graphs. Conversely, political economists were rather late to embrace the use of statistical data in their field of study.
The armchair
In Victorian times, the political economist was very much like a private detective: a skilled investigator who had learned to put his talents for observation to good use.
Arriving at the scene of a crime, the political economist would round up the usual suspects and interrogate them. Drawing out relevant bits of information, he would attempt to make associations between them, always on the lookout for necessary connections and inconsistencies. What especially excited him was searching for possible motives, and finding the one that best fit the crime. For he knew, as did any private detective worthy of his profession, that he was in the business of generating motives; he was consumed by a fascination for the dark and self-interested side of human nature.
The political economist often recorded his observations in a duodecimo-sized notebook – large enough to contain handwritten text, small enough to carry around at all times (fig. 1). On the right-hand page, it was common practice to record numerical facts, notes from conversations, excerpts from other texts, and personal experiences. Commentaries, references to other sources, noted discrepancies, and general insights were then added to the left-hand page. In this manner, the literary space of the notebook became an epistemic tool that combined observation, reflection and conjecture to infer fundamental human principles.

Figure 1. Fragment from John Elliot Cairne’s notebook on the state of Ireland, 1864
In time, when the fieldwork was done, the political economist retired to his study to evaluate his material, to sift through things and decide what was relevant. This is where the armchair comes in (fig. 2). In an effort to refine his ideas, a second and sometimes even a third version of his notes might be prepared. It was only when he had succeeded in untangling the inherent complexities of the social world, when economic and historical events could be traced to motives of human action, that the political economist was satisfied that his case was solved.

Figure 2. John Stuart Mill, circa 1858
To the extent that such fundamental insights into human behaviour escaped mathematicians and their statistical tables, political economists of the Victorian era considered both – the mathematicians and their tables – with equal quantities of suspicion and disdain.
A nineteenth-century approach to science
Graphs started to be used in political economy during the eighteenth century by William Playfair, a Scottish engineer.
In a 1785 publication entitled The commercial and political atlas: Representing, by means of stained copper-plate charts, the exports, imports, and general trade of England, at a single view, Playfair invented several types of diagrams, including line, area and bar charts (fig. 3). Later, in 1801, he also invented pie charts and circle graphs, which are used to show part-to-whole relationships.
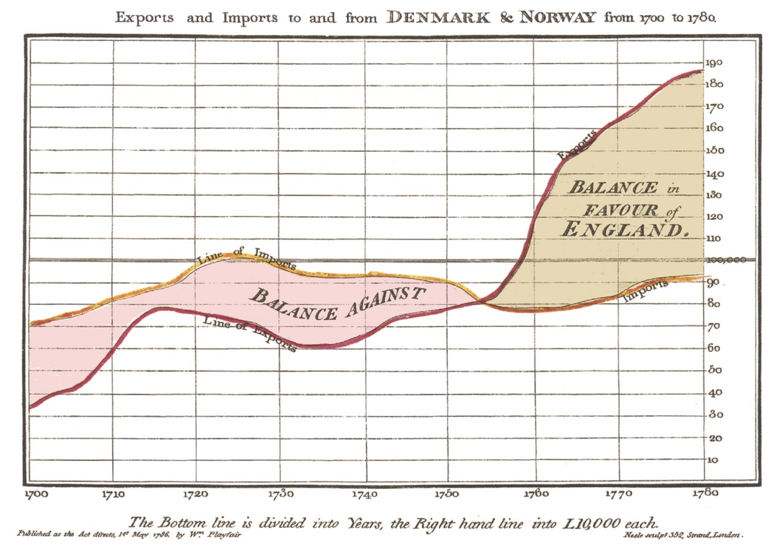
Figure 3. William Playfair, ‘Exports and imports to and from Denmark and Norway from 1700 to 1780’, in The commercial and political atlas, 1786
However, diagrams were not to be seen again in the literature for over half a century.
It was around the 1830s, as the use of statistics intensified, that interest in graphical means of expression revived and started to appear in scientific texts and, to a lesser extent, in works of social and historical analysis.
Yet, when Jevons started producing economic graphical representations from the 1860s onward, he was highly criticized for doing so by his fellow political economists.
It was mainly through the work of Alfred Marshall in 1890 that diagrams became an accepted form for representing economic concerns. Marshall was the first to plot supply and demand curves onto one and the same Cartesian plane so they could cross at what would become known as the point of equilibrium.
It was at this moment that political economists traded the literary space of observation, reflection and conjecture – the notebook and the armchair – for the Cartesian space of statistics and mathematics.
Although political economists at first used diagrams as a means of representation and persuasion, by the end of the nineteenth century they were also used as a means of investigation and comprehension.
With the change in focus from representation to investigation and the production of knowledge, diagrams in economics also changed from a concern with shape and functional form to a focus on turning points and points of intersection.
Furthermore, while representations are static in relation to what they show and refer to – for example, a bar chart presenting statistics about the Distribution of average output in the USA metropolitan areas (fig 4) – knowledge generators have a dynamic, open-ended relation to what they can provoke.

Figure 4. Distribution of average output in the USA metropolitan areas
For example, Space relationship layout for Dorben Consulting (fig. 5) is a diagram that can be used to imagine various spatial layouts for offices and production facilities.

Figure 5. Space relationship layout for Dorben Consulting
No matter how we classify these visualizations, they all participate in a visual epistemology – they are all connected to forms of knowledge production.
Today, there are few tools as important to economic pedagogy and analysis as graphic representation. Yet the graphic language of points, lines and curves hasn’t evolved very much since Playfair introduced his charts.
In many ways, the diagrammatic techniques that were developed during the nineteenth century reflect the scientific paradigm of the time, which is to say, they participate in a process of breaking nature down into its simplest possible elements, and then trying to define rules and causal relations on how these elements interact.
Many would argue that the science of economics hasn’t evolved much since the nineteenth century either, and continues to isolate and abstract aspects of human behaviour into the simplest possible actions, and then tries to establish irreducible laws to describe them.
Normal people
Graphical forms of representation may be considered as the meeting point between statistics and geometry. Without data, we would have nothing to plot. Without geometry, we are left with discrete points that will always remain unrelated.
The collection of statistical information started in the sixteenth century, when governments and other institutions began enumerating people and their habits. However, it was during the outbreak of the plague in seventeenth-century London, when parishes recorded and published weekly reports on the number and causes of death, that this practice led to the widespread collection of data, from births and crime rates to trade volumes (fig. 6 and fig. 7).

Figure 6. Fragment from a collection of Bills of mortality: Beginning on December 27, 1664 and ending on December 19, 1665

Figure 7. Fragment from John Graunt’s work on medical statistics, Natural and political observations mentioned in a following index and made upon the bills of mortality, 1662
It was at that point that societies became statistical.
A good example is the case of suicide.
During the first half of the nineteenth century, suicide was a much-studied phenomenon. One could even say it was a fad. So when a debate erupted between France and England over which country had the most suicides, a massive enumeration ensued. The result, published in 1822 by Jean-Pierre Falret, was a dissertation on hypochondria and suicide. In addition to observing that 1813 was a particularly bad year for Paris suicides (we’re not sure if that means there were a lot or very few), Falret provided the following list of predisposing causes: heredity, temperament, age, sex, education, reading novels, music, theatrical performances, climate, seasons, masturbation and idleness.
More importantly, as the figures on suicides became public, along with other forms of ‘social deviancy’, mathematicians pored over them, and as they did, they were amazed at their regularity. No matter how they sliced the data – by education, wages, religion or nationality – they were able to observe ‘invariable laws’ regarding their relative frequency. They could not help but wonder how it was that, when regarded en masse, phenomena that appeared to be the result of free will and a very complex set of social circumstances could become so predictable.
In the process, a new type of law came into being: the law of probability. The law of probability had connotations of normalcy and deviations from the norm, and as such, it had direct consequences on people and their behaviour (fig. 8).

Figure 8. Distribution of performance in a plant where methods have not been standardized
If the Enlightenment attempted to understand and define something called ‘human nature’, by the nineteenth century thinkers became obsessed with something called ‘normal people’.
The interesting thing about statistical laws concerning human behaviour is that they are both inexorable and self-regulating: people are normal if they conform to the central tendency.
Those at the extremes are considered pathological.
Consequently, most of us try to make ourselves as normal as possible, which, in turn, affects that which is considered normal. Furthermore, enumeration requires categories, and defining these new classes of people for the purposes of statistics has an impact on the way we conceive of ourselves and of others.
Each number equals one inhalation and one exhalation
It is also at the end of the nineteenth century that the concept of objectivity and scientific methods of measurement were introduced to study human productivity; an event which had a tremendous effect on the way labour has been conceived, measured and designed ever since.
The doctrines of ‘work science’, ‘Fordism’, ‘Taylorism’ and ‘scientific management’ all represent particular versions of the attempt to find positivist and scientific resolutions to the question of production. Although they differ on many levels, what they share is a commitment to ideas of rationalization in addition to technological and social efficiency (fig. 9).

Figure 9. Desola Gantt chart
The economic notion of efficiency, as the ratio of output to input, became the unquestioned rationality behind the new disciplines directed towards labour activities and labour relations.
Today, notions of economic efficiency and objective measures of work extend the original approaches of the late-nineteenth century into every area of labour, technological efficiency and business organization (fig. 10). Diagrams, graphs, time and motion studies, tables, timelines, flow charts and bar charts still play an important role in the propagation of these ideas and the day-to-day evaluation of worker performance.

Figure 10. Postinjury productivity losses – Absentee and earning effects
In graphs, labour is analyzed and broken down into units, while new methods for the management of time, communication and workflows are sketched. In the process, abstract measurements transform complex ideas about human behaviour into comparable data, and labour becomes an abstraction that reduces the specificity and meaning of concrete labour to numerical units; in other words, to labour in general.
It is this abstraction, from the concrete and particular to the general, which allows one to grasp labour quantitatively. Through this process, abstracted working activities can then be translated into a common medium – numbers – which can be compared and through which relations can be established. The capacity of graphs to make the relations between elements visible was precisely what made them fit for the task.
In Frederic S. Lee’s book, The human machine and industrial efficiency, published in 1918, we see a diagram charting the Output among men polishing metal on a ten-hour shift with no rest breaks except for lunch (fig. 11). The graph reveals the decline of productivity over time and constitutes a classic example of management scientists’ interest in maximizing productivity through optimal rest break schedules. With the word ‘LUNCH’ printed in the middle at the bottom, we can appreciate how the graph sought to visually communicate the temporality of the experiment.
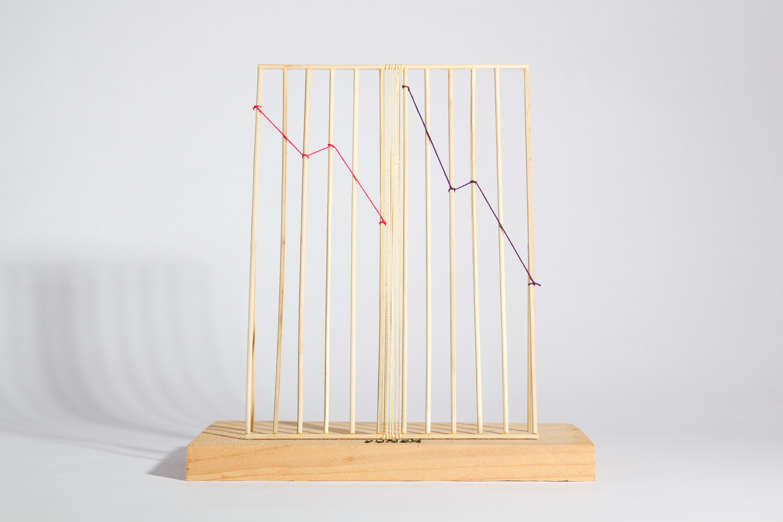
Figure 11. Output among men polishing metal on a ten-hour shift with no rest breaks except for lunch
Developed in the late-nineteenth century, the photographic study of motion served as a basis for the use of still and moving images in the analysis of human movement within industry. These approaches to work measurement were developed by Frederick Taylor, Henry Ford, and Frank and Lillian Gilbreth in the first decades of the twentieth century in the context of an industrial economy. They are still widely used today.
Between 1910 and 1924, the Gilbreths devised multiple ways to use photographic images in factories and other places of work to determine the most efficient methods of operation or ‘the best way.’ Their time and motion studies, also called ‘chronocyclegraphs’, were realized by capturing the movements of workers with long photographic exposures. The motion ‘paths’ recorded by the camera were traced by attaching tiny lamps to the body of the worker. The images could then be translated into graphics such as Two cycles on drill press (fig. 12) and Natural movement of the hand / The shortest path after operator has been trained. These graphs were then analyzed by engineers so they could identify inefficient gestures and design the most time-efficient movements to be taught and used by all workers.

Figure 12. Two cycles on drill press
Flow charts, which are endemic today in management and organization analysis, also appeared during the early part of the twentieth century. The way flow charts reduce activities, processes and complex actions into simplified workflows make them especially suited to the needs of management and administration. Because of its ability to present chronological sequences, the chart is instrumental in developing a plant layout or for improving the design of existing workspaces. Diagram of the revised layout of a group of operations (fig. 13) was produced to improve production by studying the layout of operations in a rifle factory.
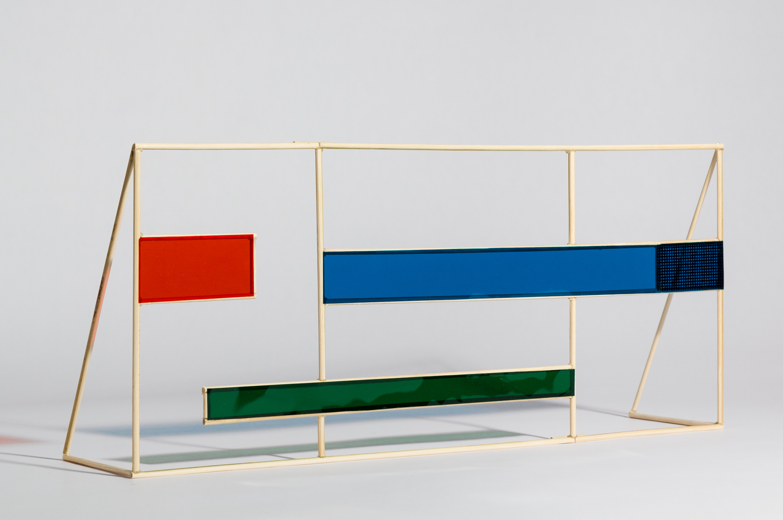
Figure 13. Diagram of the revised layout of a group of operations
Other diagrams, such as Organization chart showing the influence of methods, standards and work design of the operations of the enterprise (fig. 14), reduce the entirety of relationships within an enterprise to discrete chunks of activity, workflows and hierarchical positioning. Organization charts are very clear and visually pleasing. That’s why they are used: because they look nice on paper or on a PowerPoint slide. Generally, they’re not based on hard data, and they’re usually put on paper in a way that ‘feels’ best, and what ‘feels’ best is always whatever ‘looks’ best. As one young art critic put it: ‘Whole organizations reorganized, wages set, instructions written, based on sticks dipped in lavender and mint green food colouring?’.

Figure 14. Organization chart showing the influence of methods, standards and work design of the operations of the enterprise
One of the most famous experiments in industrial history took place at Western Electric’s factory at Hawthorne, near Chicago, between 1927 and 1933. The experiment involved changes in physical working conditions and work requirements, changes in management and supervision, and changes in the social relations of workers. Surprisingly, in all cases, productivity improved whenever a change was made. For instance, productivity improved when lighting in the workroom was augmented, but also when it was dimmed again. This became known as the ‘Hawthorne Effect’ – a reaction in which individuals modified their behaviour in response to their awareness of being observed. The graph titled Worker productivity trends in periods 1 to 24 of the first relay experiment at Hawthorne (fig. 15) presents a statistical analysis of the productivity for the 257 workweeks of the experiment.

Figure 15. Worker productivity trends in periods 1 to 24 of the first relay experiment at Hawthorne
From the latter part of the twentieth century to the present, what constitutes human productivity and how it can be measured have shifted as economies have gone through a series of transformations that have modified the nature, form and organization of labour. Amongst these transformations is a shift from the mass production of identical products to delocalized and more flexible manufacturing processes, as well as a shift to service and knowledge economies. While Fordist models considered labour as the harnessing of ‘manpower’, post-Fordist models necessitate the mobilization of not only logical and technical capacities, but also of the affective and communicative resources of the worker.
Changes in the use and organization of workplaces reflect some of these transformations. They are especially visible in the design of offices. In Coffee point placements at Novartis Research Facility (fig. 16), we see an architectural plan of one of the edifices in Novartis’s new campus in Basel, which is entirely dedicated to the emplacement of ‘coffee points’ on the different floors of the building. The plan reveals how architecture itself is designed to respond to the need for fostering collaboration and communication between workers in a knowledge economy.

Figure 16. Coffee point placements at Novartis Research Facility
To sketch Circulation route, projected teams and total workplace at Google Berlin (fig. 17), architects worked with management consultants to design flows in the workplace that would be conducive to mobility and communication between employees from different departments while creating identification within teams of workers.

Figure 17. Circulation route, projected teams and total workplace at Google Berlin
And finally, the last sculpture is called Flow diagram of the old layout of a group of operations (fig. 18). These sculptures have recently been exhibited in New York, where the same art critic quoted above visited the exhibition with a former management consultant, named Hannah. This is what Hannah had to say about this sculpture:
This is meant to visualize how the factory floor would be used by each worker. In a perfect world, none of these strings would overlap – each person would have a single route, which was never doubled back on, and they wouldn’t overlap with those of another worker. If you have machinery involved, every time these lines cross, there’s the possibility of error, or injury. You see this green guy here, he’s just fucking around, his life sucks.

Figure 18. Flow diagram of the old layout of a group of operations
The fly
It was mentioned earlier that graphical forms of representation may be considered as the meeting point between statistics and geometry.
But there is a third element without which no data may be plotted and no geometry may be calculated. This third element is space.
The concept of ‘space’ can denote the physical or temporal absence that exists between two objects or events, like the distance between two people, or the silence between two words.
It may also denote the everythingness that surrounds us, like when we look up and appreciate the vastness of the sky.
When fragmented into smaller bits, space has the capacity to retain the inherent spaceness of the original: erecting a wall gets you two spaces where before there was one.
In this way, space behaves more like a monad than an atom: it has no parts (although we may speak of a part of a space).
By placing an object in space, it is possible to create an indefinite number of new spaces: the space within the object, behind the object, under the object, and so on. Space defies arithmetic: by adding something to space, it multiplies.
Descartes is reported to have invented coordinate space while lying in bed one morning imagining how to communicate the movements of a fly he watched ambulating on the ceiling.
His solution, to describe the fly’s position relative to its distance from the walls of the room, amounted to reducing the total space occupied by the insect to a single dot.
What he gained in precision, he lost in accuracy.
He also lost the fly.
acknowledgement
Artworks pictured in figures 4-5 and 8-18 by Richard Ibghy and Marilou Lemmens from the series Each number equals one inhalation and one exhalation (2016-17).
Richard Ibghy and Marilou Lemmens are artists who live and work in Durham-Sud, Quebec, Canada. Richard and Marilou have published four artist’s books and their writings have been published in books, catalogues, and magazines. A comprehensive monograph about their practice as well as a book dedicated to one of their works, The prophets, will be published in 2020. They were awarded the Prix Giverny Capital in 2019 and the Research Fellowship of the Grantham Foundation for the Arts and the Environment in 2020.
Website: http://www.ibghylemmens DOT com/index.html
Email: richardibghy AT gmail.com
Email: mariloulemmens AT gmail.com




